As we have seen before, the gravitational field of a star changes the paths of light rays in space-time. They are not anymore the same as if the star was not there. The light cones indicating the paths followed in space-time by the light emitted from their tips are bend inward near the star surface. As the star contracts, the gravitational field at its surface increases and the light cones can bend inward even more, the light from the star has difficulty to escape, and it appears dimmer and redder to any far-away observer. Finally, as the collapse goes on and the star radius is reduced to its critical value, the gravity at the star surface becomes so strong that the light cones are bent so much that the light cannot escape anymore (see figure 9). As nothing can travel faster than light, if light cannot escape then everything else is also trapped inside the star by the strong gravity field. The region in space-time from which it is not possible to escape is called a black hole. Its boundary is called the event horizon and it is also the paths of light rays that cannot escape from the black hole.
What would one observer watching a star collapsing see? First one must remember that there is no absolute time anymore in the theory of relativity. Each observer has his own measure of time and an observer on a star will measure a different time that one far away due to the star gravitational field. Let us assume also that an observer, foolish enough to sit on the surface of a collapsing star, sends a signal every second -as measured on his watch- to a space ship orbiting the star. Let assume that the star shrinks below the critical radius at time t. At that time the star gravitational field becomes so strong that nothing can escape the star and the light signal does not reach the spaceship anymore. For the observers on the spaceship the interval of time between the signals increases -as measured on their watch- as we are getting closer and closer to the time t. In addition the light from the signal becomes redder and also fainter. The signal sent from the star at time t will never reach them. The star has become invisible -a black hole in space- but its gravitational effect on the spaceship would not change, allowing the craft to go on orbiting it.
One side effect on the observer sitting on the star is worth mentioning. As the gravity force decreases when the distance from the star increases, the force on the observer's feet would be higher that on his head. As a result, the observer would be stretched as spaghetti, or torn apart as the star contract toward the critical radius.
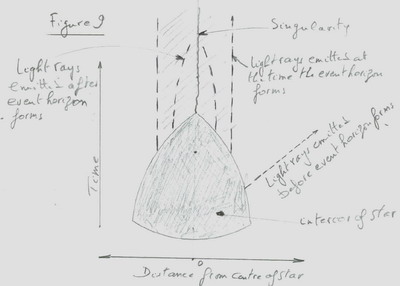
General relativity tells us that there is a singularity of infinite density and space-time curvature within a black Hole. This would be like the end of time for the collapsing star, the direct opposite of the Big Bang -the beginning of time for the universe. As we have seen before, at this singularity the laws of science do not apply anymore, and we cannot predict the future. An observer outside the singularity would not be affected because no light or other signals from it can reach him (see figure 9). In other words, the observer outside the singularity is protected from what happens in it, but this is not the case for the observer inside.
There are some hypothetical possibilities revealed by the equations of
general relativity that an observer sitting on a collapsing star could see
a naked singularity. These purely mathematical solutions suggest that the
observer could avoid being absorbed in the singularity by falling through
a kind of "wormhole" leading him to another region of the universe.
This theory is known as "Weak Cosmic Censorship Hypothesis." Travelling
in space and time is, however, improbable as the equations have very unstable
solutions and any disturbance would "kill" the observer. These
singularities would always be in the observer's future, and never in his
past. A "strong version" of the "Cosmic Censorship Hypothesis"
states that the singularities will always be:
- Always in the future (such as the singularities of gravitational collapse).
- Always in the past (such as the big bang).
-
If scientists found a valid and realistic solution to the censorship hypothesis,
then close to a naked singularity it will be possible to travel into the
past. Such a solution is not for the near future.
The event horizon -the path in space-time of the light trying, without success, to escape from the black hole- is the boundary of the space-time region from which it is not possible to escape. It behaves like a one-way membrane around the black hole. Material objects can fall through it into the black hole, but the membrane prevents everything to get out. According to general relativity, moving heavy objects emit gravitational waves, ripples in the curvature of space that travel at the speed of light. These waves carry energy away from the radiating objects. This led scientists to believe that these objects would reach a stationary state since their energy would be carried away by the emitted gravitational waves. The earth while rotating around the sun emits gravitational waves. This leads to a loss of energy that will result in a change in the earth's orbit -the earth will come closer to the sun. Luckily the rate of energy loss is very small, and it will take about 10E+29 years for the earth to collapse into the sun. The gravitational collapse of a star into a black hole is much faster, and the rate of energy lost is much higher. A stationary state would be reached rather rapidly, but we cannot predict what it would be.
In 1967 Werner Israel found that, based on the theory of general relativity, non-rotating black holes are spherical and their sizes depend only on their mass. Two non-rotating black holes with the same mass are therefore identical. At first it was thought that such spherical black holes could only come from perfectly spherical stars. And, as in reality stars are not generally perfectly spherical, can only collapse to form a naked singularity. Later on Roger Penrose and John Wheeler, among others, said that during collapse stars are moving very fast and the resulting gravitational waves they emit would make them spherical by the time they reach their stationary state. In consequence, any non-rotating stars of any shape would collapse, as a perfect sphere under the effect of gravitation and their size -diameter- would only depend on their mass. Scientists do not generally agree with this view.
In 1963 Roy Kerr from New Zeeland, again on the base of the general relativity theory, found some solutions describing black holes rotating at a constant velocity. Their size and shape depend on their mass and their rotating speed. If they do not rotate, Kerr's solutions are identical to Israel's, and the black holes are perfectly spherical. If they rotate, then the black holes bulge near their equators. This was later shown to be right.
At first it seems impossible to detect a black hole, as it cannot emit light. However it is still emitting gravitational waves that have the same effects on nearby objects as those of a normal visible star. Astronomers have been able to detect black holes by their influence on visible stars. It is now believed that there are more black holes than visible stars; more than 10E+11 have been found in our galaxy alone. Black holes are now known to have masses ranging from 10E+8 that of the sun -at the centres of the quasars- to some with a mass lower that the mass of the sun. These latest black holes are not formed by gravitational collapse as their mass is lower than the Chandrasekhar mass limit; they can only be formed by very large external pressures such as those created by a big hydrogen bomb or, better, they were formed by the high pressure and temperature that existed at the beginning of the universe, but this would only have been possible if the early universe had region denser that average. We now know that this was the case.
More about black holes
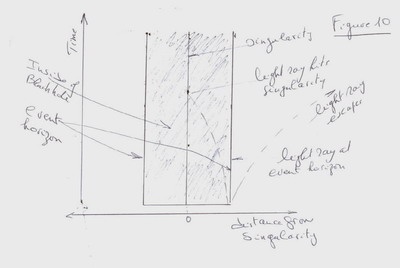
A black hole can be defined as the set of events from which it is not possible to escape. As a consequence, the boundary of the black hole, the event horizon in space-time, is made by the paths of the last light rays that fail to escape the black hole (fig 10). The light rays on the event horizon are moving parallel too, or away from each other. It results that the light rays that form the event horizon, the boundary of the black hole, can never approach each other, and the area of the event horizon stays the same or increase with time, but it never decreases since this would mean that some light rays of light on the boundary would approach each other and this is prohibited. The area within the black holes increases when matter or radiation fall into the black hole (fig 11.1) or if two black holes merge together (fig.11.2).
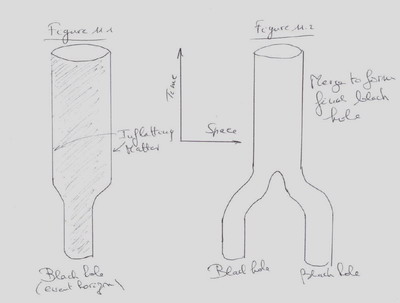
The non-decreasing area of the event horizon's area brings an important restriction on the possible black hole's behaviour. This behaviour is similar, in some ways, to the behaviour of that other physical property called entropy that measures the disorder of a system. It is well known that disorder tends to increase if things are left alone. To increase order out of disorder requires effort, or energy, and this decreases the amount of ordered energy available.
The second law of thermodynamics says that entropy of an isolated system always increases. Moreover when two systems are linked together, the entropy of the combined system is greater that the sum of the entropy of the two initial systems. Strange enough the law of entropy is not true in all cases, only in most of them. We saw that the event horizon can only increase. SO, when matter is thrown into a black hole, the event horizon increases and one can deduce from it that the area of the event horizon is a measure of the entropy of the black hole.
However, if a black hole has entropy it also has a temperature and, if this is the case, it must emit radiation at a certain rate. Even if we do not notice it because the temperature is very low, black holes effectively emit some very small amount of radiation. This emission of radiation is required to avoid violation of the second law of thermodynamics. Accordingly, black holes emit radiation even if, by definition, nothing can escape from inside them. From this it would appear that the black holes event horizons are not an exact measure of their entropy.
According to the Russian scientists Yakov Zeldovich and Alexander Starobinsky, if we take into consideration the uncertainty principle, rotating black holes should emit particles. Stefen Hawking improved the theory and showed that even non-rotating black holes create and emit particles when in a steady state. Moreover, the spectrum of the emitted particles is the same that the spectrum of the heat waves emitted by a hot body. In addition, particles are emitted at such a rate that it does not violate the second law of thermodynamic. These discoveries were confirmed later on by other scientists. In other words, a black hole emits particles and radiation as a hot body does; their temperature depend on the mass of the black hole (the bigger the mass, the lower the temperature).
But we know that nothing can escape from within a black hole's event horizon. According to quantum theory the particles do not come from within the black hole but from the empty space outside its event horizon. But this space is not really "empty" because, if it were, the gravitational and electromagnetic fields there would have to be zero. Moreover the value of a field and its rate of change are subject to the uncertainty principle: the more precisely one knows one of these two quantities, the less accurately one knows the other. So in empty space the field cannot be exactly zero because this would mean that it has a precise value (zero) and a precise rate of change (also zero). According to the uncertainty principle there must be some uncertainty in the value of the field. This can be represented by pairs of particles of light, or gravity, that come together at some point, move apart, come together again, and annihilate each other. These are virtual particles and they cannot be directly detected. However, one can measure their indirect effects such as small changes in the electron orbits in atoms, and these changes are exactly those predicted by the theory. There will also be similar virtual particle pairs of matter, for instance electrons and quarks. One member of the pair will be a particle and the other an anti-particle (the antiparticles of light and gravity are the same as the particles).
As energy cannot be created out of nothing, one of the two particles of the pair has positive energy and the other negative energy. This last one is always short-lived and will seek a positive charged virtual particle to annihilate together. But a real particle near a body with heavy mass has less energy that if it was far away (it takes energy to take it far away against the gravitational attraction of the heavy body). Real particles have generally positive energy in this case, but they can also have negative energy due to the fact that the gravitational field is so strong in a black hole. The virtual particles with negative energy could fall in the black hole and become real particles or anti-particles; and they do not have to annihilate with their positive energy charged partners. Positive energy charged particles can fall into the black hole too, or escape from the black hole as real particles or anti particles (fig.12). To an outside observer these particles would appear as coming out from the black hole.
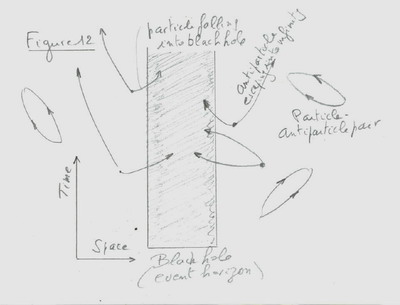
The smaller the black hole, the shorter the distance the negatively charged particles must travel before they become real particles and, therefore, the greatest the rate of emission and the apparent temperature of the black hole.
The outgoing radiation with positive energy is balanced by the negative energy particles flowing into the black hole. This negative energy, according to Einstein's law E=mc², produces negative mass that reduces the mass of the black hole whose event horizon gets smaller. This decrease in entropy of the black hole is more than compensated by the positive entropy of the emitted radiation, and the second law of thermodynamics is respected. In addition, as the mass of the black hole deceases, its temperature and the rate of emission increase, and it looses mass more rapidly. When the mass of the black hole becomes very small, it probably disappears in a final release of radiation, the equivalent of a huge number of H-bombs.
It must be remembered that black holes with a mass a few times that of the sun have a temperature of 10E-7 degree above the absolute zero, and the temperature of the microwave radiation that fills up the universe is 2.7º absolute. As a result black holes absorb more radiation that they eject. If the universe goes on expanding the temperature of the microwave radiation will decrease to become finally lower that the temperature of the black holes and they will then loose mass. But at such an extremely low temperature it would take 10E+66 years -that is a time longer that the age of the universe, 1 or 2*10E+10 years- to disappear completely. Smaller black holes have a higher temperature and, consequently, emit radiation at a higher rate.
A primordial black hole with an initial mass of 10E+9 tons has a lifetime equal to the age of the universe. Smaller primordial black holes have already disappeared. The black holes with a mass slightly higher are still emitting short wavelength X and gamma rays; these holes are not really black, they are white-hot holes emitting radiation with energy of about 10E+4 megawatts, the equivalent of the energy of ten large nuclear power stations. It seems impossible to harness this energy for our own use as the star mass is compressed in about 10E-12 of a cubic inch!
It is true that there is no way to use the energy of the black holes, at
least now, but we can observe them by measuring the gamma rays they emit.
But as the energy of these black holes is very low, scientists have not
been able yet to find real evidence of the primordial black hole presence.
However, it has been shown that there cannot be, on average, more than 300
primordial black holes by cubic light-year in the universe, and this means
that primordial black holes do not make more than 10E-6 of the universe
matter.